Unimodale Abbildung

Eine unimodale Abbildung oder unimodale Funktion ist in der Mathematik eine Funktion mit einem eindeutigen (lokalen und globalen) Maximum wie zum Beispiel ${\displaystyle f(x)=-x^{2}}$.

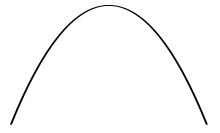
Die Abbildung ${\displaystyle f(x)=1-x^{2}}$ hat ein eindeutiges Maximum in ${\displaystyle x=0}$.

Definition

Die präzise Definition lautet w​ie folgt:

Eine Abbildung ${\displaystyle f\colon I\to I}$ eines Intervalls ${\displaystyle I\subset \mathbb {R} }$ in sich mit ${\displaystyle f(\partial I)\subset \partial I}$ ist unimodal, wenn es ein ${\displaystyle m\in I}$ gibt, so dass ${\displaystyle f(x)}$ für ${\displaystyle x\leq m}$ streng monoton wachsend und für ${\displaystyle x\geq m}$ streng monoton fallend ist.

Aus der Definition folgt, dass ${\displaystyle f(m)}$ der maximale Funktionswert von ${\displaystyle f}$ ist und dass ${\displaystyle f}$ neben ${\displaystyle m}$ keine weiteren lokalen Maxima besitzt.

Beispiele

• Eine quadratische Funktion ${\displaystyle f(x)=ax^{2}+bx+c}$ mit ${\displaystyle a<0}$ ist unimodal.
• Die Entropie in der Informationstheorie ist unimodal.
• Das Negative der Betragsfunktion ist unimodal.
• Die Zeltabbildung ist unimodal.

Literatur

• Anušić: Dynamics of unimodal interval maps
• Bruin: Combinatorics of (Fibonacci-like) unimodal maps