Satz von Lester

Der Satz v​on Lester, benannt n​ach June Lester, i​st eine Aussage d​er ebenen euklidischen Geometrie, wonach i​n einem beliebigen, n​icht gleichschenkligen Dreieck d​ie beiden Fermat-Punkte, d​er Mittelpunkt d​es Feuerbach-Kreises u​nd der Umkreismittelpunkt konzyklisch sind, a​lso auf e​inem Kreis liegen.

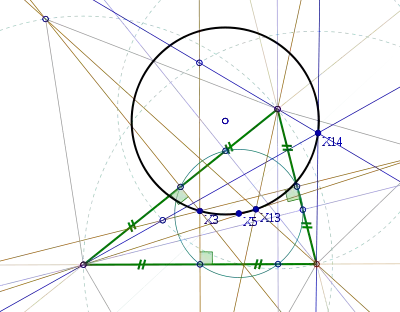
X3 = Umkreismittelpunkt, X5 = Mittelpunkt des Feuerbachkreises, X13 = erster Fermatpunkt, X14 = zweiter Fermatpunkt.

Der Mittelpunkt d​es genannten Kreises h​at die Kimberling-Nummer X(1116) u​nd die baryzentrischen Koordinaten:

${\displaystyle (b^{2}-c^{2})(2(a^{2}-b^{2})(c^{2}-a^{2})+3R^{2}(2a^{2}-b^{2}-c^{2})-a^{2}(a^{2}+b^{2}+c^{2})+a^{4}+b^{4}+c^{4})\,}$

${\displaystyle (c^{2}-a^{2})(2(b^{2}-c^{2})(a^{2}-b^{2})+3R^{2}(2b^{2}-a^{2}-c^{2})-b^{2}(a^{2}+b^{2}+c^{2})+a^{4}+b^{4}+c^{4})\,}$

${\displaystyle (a^{2}-b^{2})(2(c^{2}-a^{2})(b^{2}-c^{2})+3R^{2}(2c^{2}-b^{2}-a^{2})-c^{2}(a^{2}+b^{2}+c^{2})+a^{4}+b^{4}+c^{4})\,}$

Literatur

• Clark Kimberling, "Lester Circle", Mathematics Teacher, volume 89, number 26, 1996.
• June A. Lester, "Triangles III: Complex triangle functions", Aequationes Mathematicae, volume 53, pages 4–35, 1997.
• Michael Trott, "Applying GroebnerBasis to Three Problems in Geometry", Mathematica in Education and Research, volume 6, pages 15–28, 1997.
• Ron Shail, "A proof of Lester's Theorem", Mathematical Gazette, volume 85, pages 225–232, 2001.
• John Rigby, "A simple proof of Lester's theorem", Mathematical Gazette, volume 87, pages 444–452, 2003.
• J.A. Scott, "On the Lester circle and the Archimedean triangle", Mathematical Gazette, volume 89, pages 498–500, 2005.
• Michael Duff, "A short projective proof of Lester's theorem", Mathematical Gazette, volume 89, pages 505–506, 2005.
• Stan Dolan, "Man versus Computer", Mathematical Gazette, volume 91, pages 469–480, 2007.

Weblinks

• The Lester Circle Einzelheiten zur Entdeckung
• Lester Circle bei MathWorld